
|
|
|
|
Research Summary
I am an associate professor of Electrical & Computer Engineering at The University of Texas at Dallas and director of the NeuroSpinCompute Laboratory.
My research objective is to invent, design, and analyze novel logical and neuromorphic computing paradigms that exploit nanoscale phenomena to achieve greater capabilities than conventional CMOS architectures.
In sharp contrast to other approaches for beyond-CMOS and spintronic computing, the central theme of my research is to ensure that individual switching elements can be cascaded and integrated in efficient large-scale information processing systems.
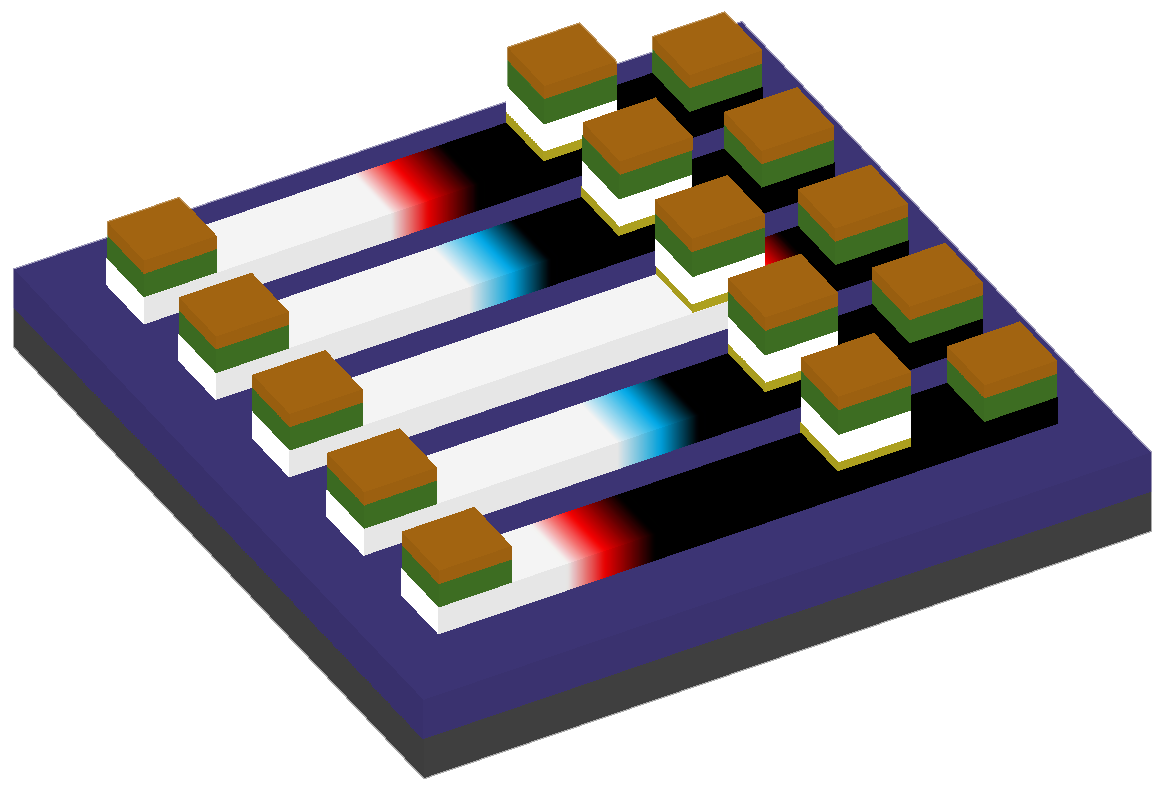 |
Magnetic domain wall neurons and synapses emulate the behavior of neurobiological elements through manipulation of magnetic domain walls.
The proposed artificial neurons are the first that intrinsically provide the leaking, integrating, firing, and lateral inhibition capabilities without any additional devices or circuitry.
This structure is used to perform handwritten digit recognition with 94% accuracy. |
|
This project aims to design and demonstrate an online learning circuit that leverages the stochastic switching of STT-MRAM devices to enable on-chip online learning and recognition. |
 |
 |
Emerging nanotechnologies intrinsically feature three exciting switching phenomena that can be directly applied to hardware security and trust without requiring any hardware overhead: stochasticity, polymorphism, and non-volatility. This project aims to leverage these phenomena to develop security and trust solutions based on a wide range of emerging technologies. |
|
Reversible skyrmion logic leverages magnetic skyrmions in the first nanoscale realization of conservative logic, providing a vision for energy-efficient computation.
In this system, magnetic skyrmions propagate through a two-dimensional ferromagnetic structure while performing reversible logic operations at the gate junctions.
A simple global clock enables direct cascading with the potential for scalable high-speed low-power reversible Boolean and quantum computing. |
 |
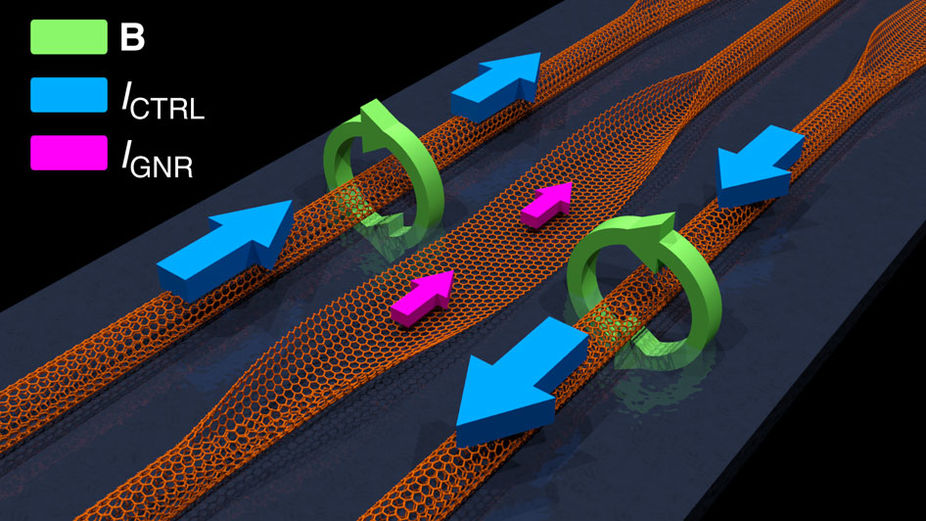
from Nature Communications 8, 15635 under CC BY 4.0 |
In all-carbon spin logic (ACSL), graphene nanoribbons (GNRs) function as spin-diodes connected by carbon nanotubes (CNTs) in accordance with spin-diode logic (SDL). The exceptional properties of low-dimensional carbon, in concert with electromagnetic wave-based signal propagation, provide the potential for Terahertz operation and a 100x improvement in power-delay product. |
|
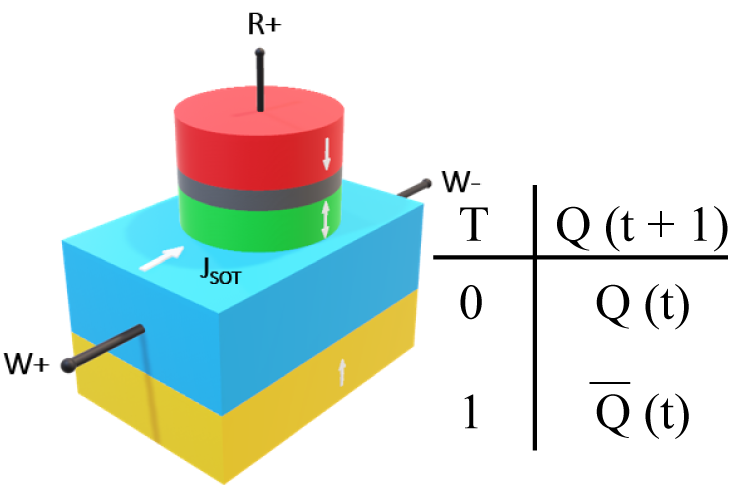
Magnetoresistive random-access memory (MRAM) promises non-volatility with SRAM-like speed and DRAM-like density and energy-efficiency. However, the efficient spin-orbit torque (SOT) switching of MRAM with perpendicular magnetic anisotropy (PMA) requires complex structures with poor tolerance of noise. To resolve this issue, toggle SOT MRAM with PMA enables robust switching with a simple device structure. |
 |
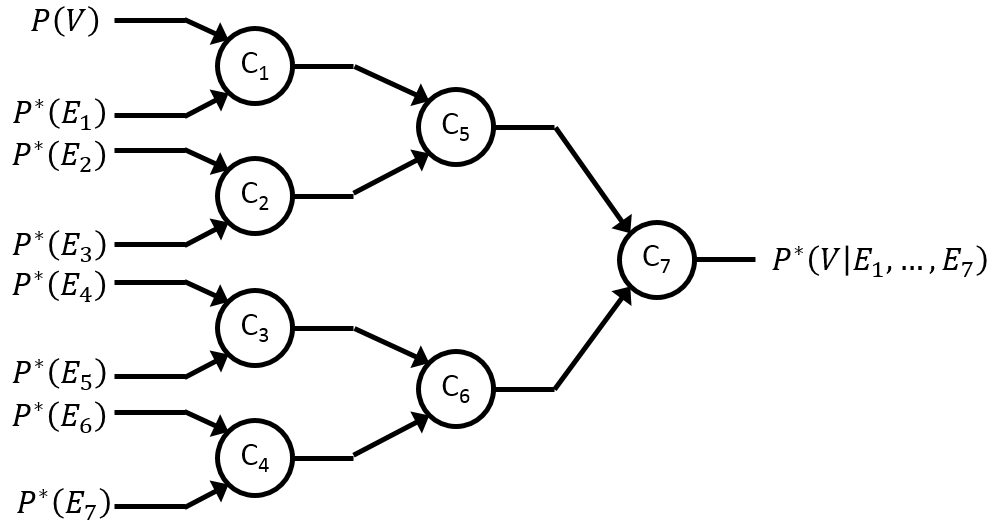 |
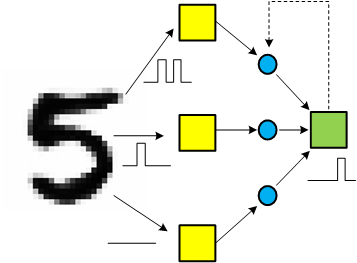
Bayesian inference is a powerful approach for integrating independent conflicting information for decision-making and robotics, performed with limited efficiency by general-purpose computers.
Excitingly, Bayesian inference can be performed extremely efficiently through stochastic computing with Muller C-elements.
This fault-tolerant circuit structure enables naive Bayesian inference with multiple orders of magnitude decrease in AEDP. |
|
The non-volatility of memristors enables stateful logic, in which bits are encoded as binary resistance states.
However, an alaysis of the required control circuit shows that when this overhead circuitry is included, stateful memristor logic is one billion times less efficient than conventional CMOS logic! |
 |
|




