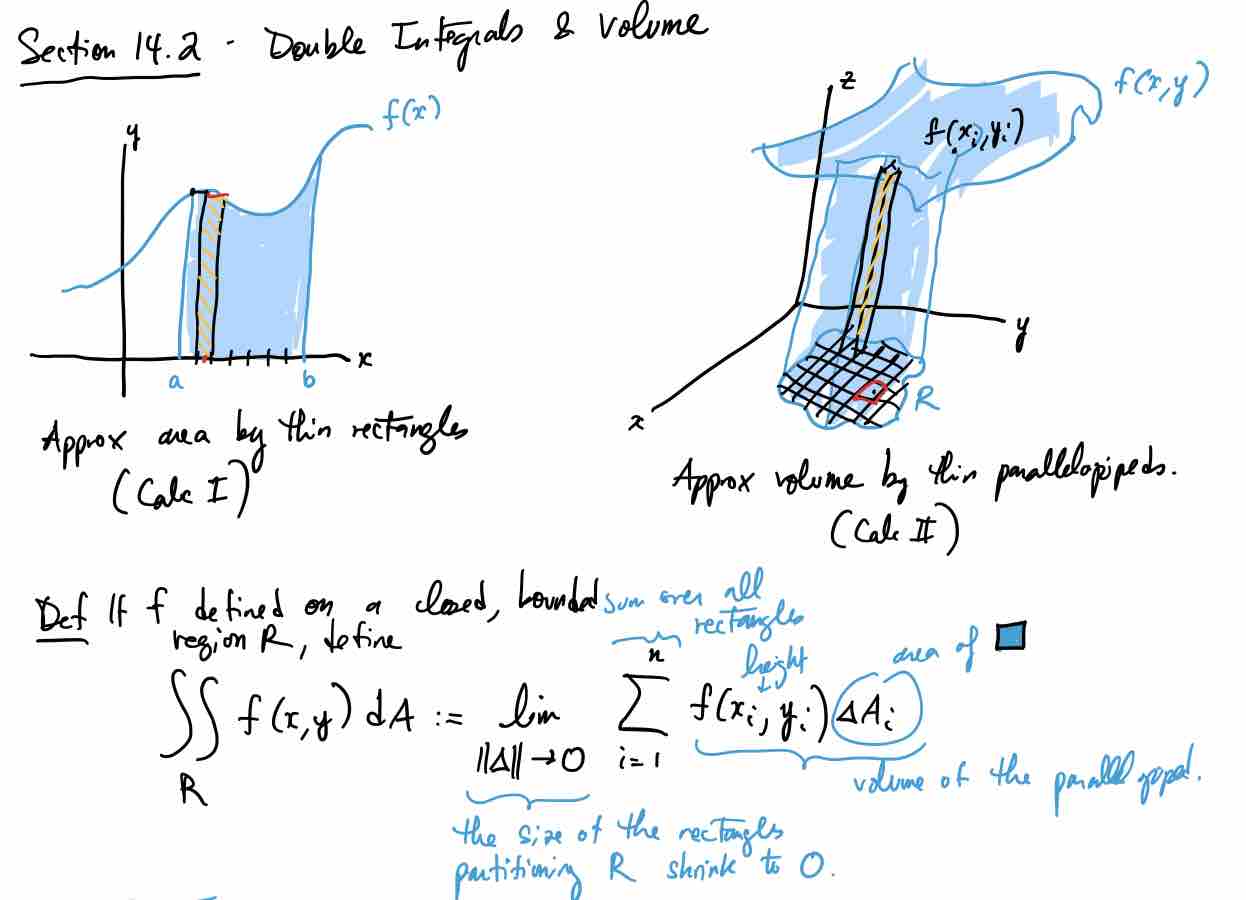
| Google Scholar | UTD Faculty Profile | ORCID |
About Me
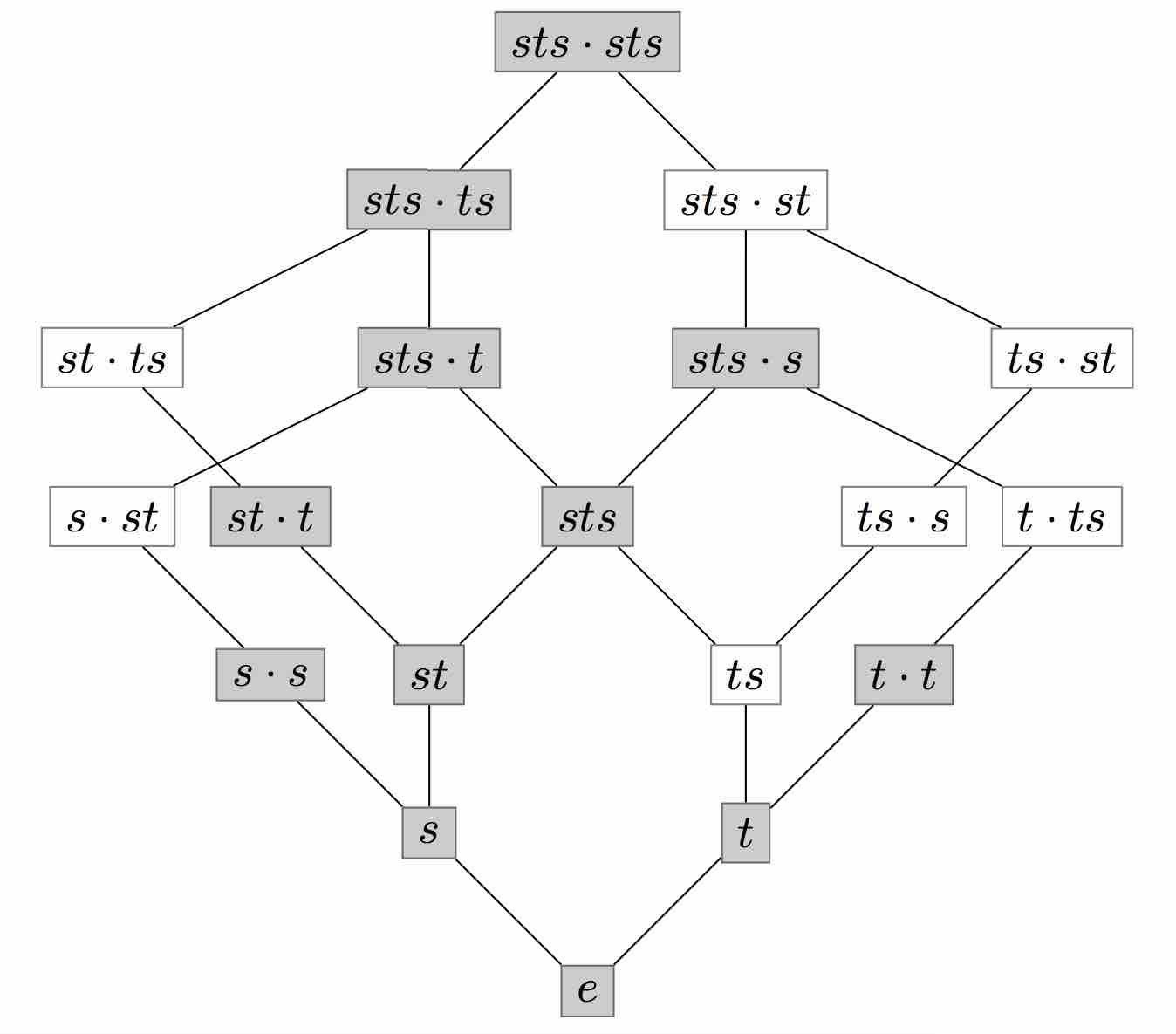
I received my Ph.D. in 2013 from the University of Minnesota, under the supervision of Dennis Stanton; the title of my dissertation was "Cataland". I then had the opportunity to spend 2+1 wonderful years at LaCIM as a postdoc—my original offer was for two years, but I was able to stay for one additional year because Hugh Thomas came to LaCIM with a Canada Research Chair and generously extended my postdoc. (Thanks to this extra year, Hugh and I were able to invert the sweep map!)
After one year at the University of California, Santa Barbara as Jon McCammond's postdoc, I started at my current tenure-track position at the University of Texas at Dallas in 2017.
I am roughly Γ() years old.
After one year at the University of California, Santa Barbara as Jon McCammond's postdoc, I started at my current tenure-track position at the University of Texas at Dallas in 2017.
I am roughly Γ() years old.