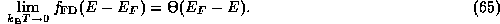
Another general approach to near-equilibrium transport is embodied in the celebrated Landauer formula [24,25,26]. This formula expresses the conductance of a system at T=0 in terms of the quantum mechanical transmission coefficients discussed above.
The Landauer formula has become the standard theoretical model by which the results of experiments on electron waveguides [3] and ballistic magnetotransport are interpreted. The presently accepted form of the Landauer formula may be readily derived from the expression for the one-dimensional tunneling current (39). In the limit of absolute zero temperature, the Fermi-Dirac distribution function (19) becomes a step function:
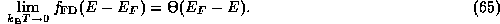
If a small bias voltage 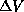 is applied to the system,
is applied to the system, 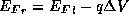 .
Then
.
Then
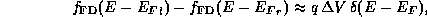
and the integral in (39) may be evaluated to obtain the conductance:
where, in the tradition of this field, the factor of 2 due to the spin degeneracy
is implied in the sum over the transverse modes (which are now termed ``channels'').
The constant  is the ``quantum of conductance,'' and is equal to
39.6
is the ``quantum of conductance,'' and is equal to
39.6 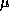 S, or its inverse is 25.2 k
S, or its inverse is 25.2 k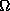 .
.
One can obtain an alternative form of the Landauer formula by considering a
different definition of the voltage drop 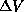 [27,28].
Equation (66) is
obtained if one defines the voltage drop by measuring the chemical potentials
deep within the respective reservoirs. In experimental terms, this corresponds to
a two-terminal measurement. In practice one often employs the Kelvin probe, or a
four-terminal arrangement, separating the current conducting from the voltage sensing
terminals. In this case it appears that the Landauer formula must be modified
because the measured
voltage drop will not equal
[27,28].
Equation (66) is
obtained if one defines the voltage drop by measuring the chemical potentials
deep within the respective reservoirs. In experimental terms, this corresponds to
a two-terminal measurement. In practice one often employs the Kelvin probe, or a
four-terminal arrangement, separating the current conducting from the voltage sensing
terminals. In this case it appears that the Landauer formula must be modified
because the measured
voltage drop will not equal 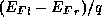 .
The problem is that, if
the transmission coefficient
.
The problem is that, if
the transmission coefficient  is nonzero, the densities of electrons
on either side of the device will not have the same values that they would have in
equilibrium. Suppose that the potential for electrons is lower in the right-hand
electrode, so the electron flow is from left to right. If the device had a
very large energy barrier, so
is nonzero, the densities of electrons
on either side of the device will not have the same values that they would have in
equilibrium. Suppose that the potential for electrons is lower in the right-hand
electrode, so the electron flow is from left to right. If the device had a
very large energy barrier, so  , the electron density due to the
states with energies E such that
, the electron density due to the
states with energies E such that 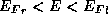 consists of two equal
parts: that due to the incident electrons and that due to the reflected electrons.
Now, if T = 1, no electrons are reflected, but the density of incident electrons
is still the same, so there is only one-half the electron density in the energy
range
consists of two equal
parts: that due to the incident electrons and that due to the reflected electrons.
Now, if T = 1, no electrons are reflected, but the density of incident electrons
is still the same, so there is only one-half the electron density in the energy
range  in the left-hand lead. Over a small energy range,
the Fermi level is
proportional to the electron density, and also the electron deficit on the left-hand
side is proportional to T, so we have
in the left-hand lead. Over a small energy range,
the Fermi level is
proportional to the electron density, and also the electron deficit on the left-hand
side is proportional to T, so we have

where 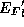 is the quasi-Fermi that would be measured in the left-hand lead.
Now the electron density which was missing from the left-hand lead appears in the
right-hand lead, raising its quasi-Fermi level. Consequently,
is the quasi-Fermi that would be measured in the left-hand lead.
Now the electron density which was missing from the left-hand lead appears in the
right-hand lead, raising its quasi-Fermi level. Consequently,

The measured voltage drop is now
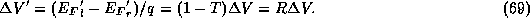
Correcting the Landauer formula (70) for the measured voltage drop leads to
which was, in fact, the form originally proposed by Landauer [24].
Which of these two forms, (66) or (70), is appropriate for a given measurement is still a subject of some debate [26]. The question is whether the experimental voltage probes are sufficiently weakly coupled to the transporting system so as to give an unbiased measurement of the local quasi-Fermi level as assumed above. To provide an explicit description of multiprobe experiments, Büttiker derived a formula for the current in each lead in a multiprobe system [29]:
where i and j index the leads. This formula is derived assuming that the current
in each lead is carried by only one channel. In this connection, one should also
note the more general formula for the multi-channel case (assuming the quasi-Fermi level
correction), derived by Büttiker
et al. [29]. If there are N conducting channels to the
left and 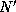 conducting
channels to the right,
conducting
channels to the right,
Because this equation is expressed in terms of the electron velocities, is also valid for non-parabolic energy band structures, as discussed previously.
Despite the attention directed toward linear-response theories, they remain severely limited in the range of physical situations which they address. The Landauer formula is only valid at very low temperatures and very small bias voltages. A finite-temperature form has been derived [28], but it is merely a restatement of the tunneling theory described in Section ii. In fact the Landauer formula in its various forms contains no physics beyond that contained in the tunneling theory. In particular, it does not deal with dissipative scattering processes within the transporting system. The Kubo formula, on the other hand, can include such processes if they are incorporated into the evaluation of the current correlation functions. Neither of these approaches is appropriate for a ``small-signal analysis'' in the engineering sense of this term. Such an analysis studies small departures from a steady-state, but typically far from equilibrium situation (a significant voltage drop occurs). The linear-response theories study small departures from equilibrium, not from a non-equilibrium state.